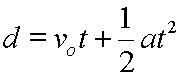A recent journal club article, the exact nature of which is irrelevant, triggered a coffee-room discussion on the subject of z scores, which although often understood in relation to Bone Mineral Density reports are otherwise a statistical challenge. In particular the difficulties in interpreting them in a meaningful way were lamented by our team.
First, we ought to ensure a clear understanding of when z scores should be used and how they are calculated. It might be most helpful to point out here that the ‘z’ simply refers to the z distribution, which is what statisticians call the normal distribution when they wish to make clinicians feel lost.
Z scores are intended for the comparison of normally distributed data which has been measured on multiple scales. Consider an example – depression in adults is measured using the Patient Health Questionnaire (PHQ-9), Hospital Anxiety and Depression Scale (HADS), Hamilton Depression Rating Scale, and Beck Depression Inventory, to name but a few. How then do we compare the results of studies of interventions for depression if the scales are different? The answer, of course, is using z scores!
Z scores are the result of converting any such data to a ‘Standard’ normal distribution, with a mean of zero and standard deviation of 1. Thus a z score represents the difference of a score from the population mean in standard deviations. So a z score of -0.5 indicates a score for depression that is 0.5 standard deviations below the mean for the scale that has been used. The z scores for the different depression scales are now comparable and can be combined to give summary data.
Conversion back to a clinically meaningful score is slightly more philosophically challenging and statistical purists might argue, inappropriate, as it is difficult to identify which scale of those you have combined you should choose to convert back into. However, if you are determined to do so and you know the mean and standard deviation of the scale you wish to convert back to, then this is simply a case of:
Mean + (z score x Standard Deviation)
One thing is certain, whether we quite understand them or not, we will continue to see z scores reported in the literature for years to come…
– Jess Morgan, University of York